A to Z of Excel Functions: The IMSQRT Function

Welcome back to our regular A to Z of Excel Functions blog. Today we look at the IMSQRT function.

The IMSQRT function

An imaginary number is a complex number that can be written as a real number multiplied by the imaginary unit i (sometimes denoted j) which is defined by its property i² = −1.  In general, the square of an imaginary number bi is −b².  For example, 9i is an imaginary number, and its square is −81.  Zero is considered to be both real and imaginary.

An imaginary number bi can be added to a real number a to form a complex number of the form a + bi, where the real numbers a and b are called, respectively, the real part and the imaginary part of the complex number.

The polar form of a complex number is another way to represent the number.  The form z = a + bi is called the rectangular form of a complex number.

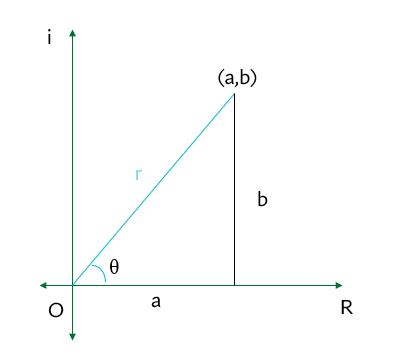
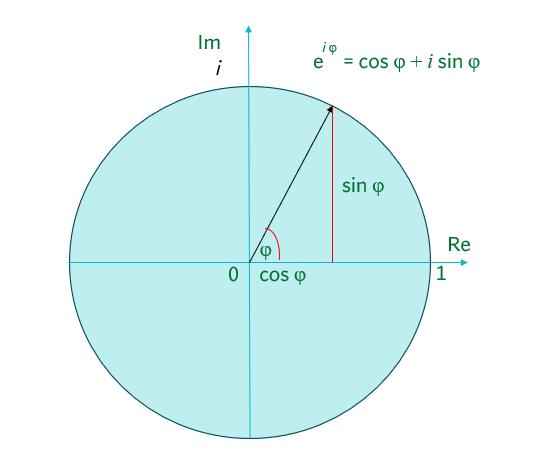
The horizontal axis is the real axis and the vertical axis is the imaginary axis.  You can find the real and imaginary components in terms of r and θ, where r is the length of the vector and θ is the angle made with the real axis.

From the Pythagorean Theorem,

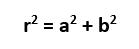
By using the basic trigonometric ratios,

Therefore, multiplying each side by r:

Therefore,

In the case of a complex number, r represents the absolute value, or modulus,

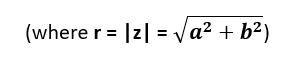
and the angle θ is called the argument of the complex number,  

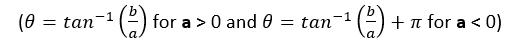
Using Euler’s Formula,

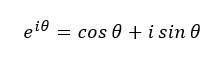
It is using this approach that the square root of the complex number x + yi may be determined:

where:

The IMSQRT function returns the square root of a complex number in x + yi or x + yj text format.  It employs the following syntax to operate:

The IMSQRT function has the following argument: 

• inumber: this is required and represents the complex number for which you want to calculate the square root.

It should be further noted that:

• you should use COMPLEX to convert real and imaginary coefficients into a complex number
• IMSQRT recognises either the i or j notation
• if inumber is a value that is not in the x + yi or x + yj text format, IMSQRT returns the #NUM! error value
• if inumber is a logical value, IMSQRT returns the #VALUE! error value
• if the complex number ends in +i or -i (or j), i.e. there is no coefficient between the operator and the imaginary unit, there must be no space, otherwise IMSQRT will return an #NUM! error.

Please see my example below: 

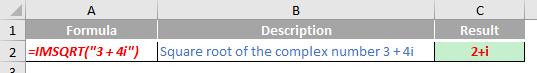
We’ll continue our A to Z of Excel Functions soon. Keep checking back – there’s a new blog post every business day.

A full page of the function articles can be found here.